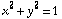and the hyperbolic paraboloid 
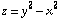on the same set of axes, and parametrize the curve C where they intersect. Verify Stokes's Theorem for the vector field F(x,y,z)=(
, 
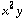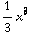, xy) and the curve C. [i.e. Show that the line integral of F along C equals the appropriate surface integral. You will need to produce your own surface.]2) Now consider the vector field F(x,y,z)=(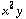
, 
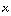, xy).a) Calculate the line integral of F along the circle parametrized by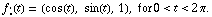
b) Calculate the line integral of F along the circle parametrized by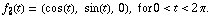
c) Calculate the surface integral of curl(F) over the surface parametrized by g(s,t)=(cos(t),sin(t),s), for 0<t<2 π and 0<s<1.
d) Explain your answers in terms of Stokes's Theorem.
3) Use Stokes's Theorem to evaluate the line integral of the vector field F(x,y,z)=(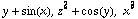
), where C is the curve parametrized by f(t)=(sin t, cos t, sin(2t)), for 0≤t≤2π.4) Consider the vector field G(x,y,z)=(x,y,z). Use Stokes's Theorem to prove, by contradiction, that G is not the curl of any smooth vector field F. [Hint: Let M be the unit sphere centered at the origin. The path integral over ∂M of every smooth vector field F equals 0 -- explain why!]