A linear family of plane cubics
This example is a linear family of plane cubic curves, which
has no base points at finite distance.
 The equation
of a curve in this family is
The equation
of a curve in this family is
y2=
x3 + x2 + bx + 2b,
where b is a parameter. The parameter values
shown in the figure are:
-
b = 0

This curve (y2 =
x3 + x2) obviously has a singularity at the
origin.
-
b = 0.05
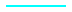
b = 0.1 
Both of these curves are nonsingular, and the
real locus is connected.
-
b = -0.05

b = -0.112 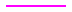
Both of these curves are nonsingular, but the
real locus is disconnected.
The defining equation of the family is obviously linear in the parameter
b, and it is easily checked that there are no base points at finite
distance.
Thus, it follows from Bertini's theorem that a general curve in this
family has no singular points at finite distance. There is a base point
at infinity, but the curves in the family happen to be nonsingular there.
We conclude that there are only finitely many singular curves in the
family, and the parameter value b= 0 corresponds to
one of them.
Which other parameter values correspond to singular curves
??
- To answer this question, we note that a plane cubic of the
form y2 = f(x) has a singular point if and only if the polynomial f(x) has a multiple root. We can determine
whether or not this happens by calculating the discriminant of f(x).
For a general cubic polynomial
f(x) = x3 + ax2 + bx + c,
the discriminant is given by the formula
D = 4a3c - a2c2 - 18abc
+ 4b3 + 27c2.
With a = 1 and c = 2b this becomes
D = 4b3 + 712 + 8b =
b(4b2 + 71b + 8b).
- So, the discrimant
is = 0 for the values b= 0,
b= -0.1134, and b= -17.64.
- The case b= 0 gives the curve shown in red,
with a node at the origin.
- The case b= -0.1134 is not explicitly shown.
It is similar to the curve shown in magenta
(b= -0.112), but with the small oval shrunk to
the single point (x,y) = (-0.719, 0). That point is the
singular point. It is a node; however we cannot see the tangent
directions because they are not real. In other words, they become
"visible" only if we allow complex coordinates and work in
C2 rather than the usual real plane
R2.
- The case b= -17.64 also is not shown.
As in the case b= -0.1134 one component of the
real curve is a single point ((x,y) = (-2.781, 0) in this case),
and this is the singular point - a node with non-real tangent directions,
as in the previous case. This curve and its close neighbors in the
family are not shown, because the scale of the drawing would have to
be larger. [Drawing to be linked at a later date. ...]
. . .
[To be continued.]
Back to the introductory
algebraic curves page.
Back to
my homepage.
 The equation
of a curve in this family is
The equation
of a curve in this family is
 The equation
of a curve in this family is
The equation
of a curve in this family is