Assignment 4 - Due Thursday 2/21/2002
Hand in the starred (*) problems.
Read: Simmons, Sections 10.6, 10.7, 10.8. We will not do 10.9, but by all means read it! Your calculators probably use a method of integrating functions numerically which is more sophisticated than the basic approach described in 10.9, but at least this section gives you some idea of what they might be doing.
Exercises:
Sec. 10.6: 1b, 6, 10, 11, 15*, 16, 20, 21, 22*, 24
Sec. 10.7: 3, 5*, 7*, 8, 9, 12, 14*, 17, 18*
Sec. 10.8: 12*, 17, 26, 39*, 43*, 96, 102
Exam on Monday February 18:
This is a 50 minute exam in class and it will be on sections 8.1-8.6, 9.4, 9.5, 10.1-10.5. Since you will be required to do a number of integrals, I have decided not to allow calculators in this exam.
There are many techniques of integration you have to remember and be able to apply, and also some basic integrals you need to have available. Because of this the integrals which appear on the back inside cover of the book will be listed on the first page of the exam, except for the integrals of un, sin u, cos u, cot u and csc u. I will not ask you any questions which require you to integrate expressions with cot and csc. The other integrals I just mentioned are so basic that I expect you to remember them. On the first page of the exam it will also be stated that
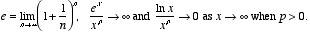
We were very quick going over section 9.7 about the hyperbolic functions, and my main purpose was simply to mention them so that at least you have seen them. I will not ask you specifically about these functions. Their use for us at the moment is that they enable us to do a type of integral whose answer can be expressed in terms of sinh-1 as on page 327. These integrals can also be done by substituting u = tan w, so that you do not need to know the hyperbolic functions at all to do the questions I might ask.
Some of the integrals we have seen how to do, such as the various kinds of trigonometric integrals get very long and complicated. It is not my intention to present you with very complicated integrals on the exam.
You need to know how to use the properties of ex and ln x etc, but not how to derive these properties. So, for example, you may be asked to evaluate limits like those on page 269 question 25 and page 277 question 22 and the limits of this kind that you have seen in class, on quizzes and in homework, most of which were more difficult than the questions I have just mentioned. You should give correct mathematical manipulation reducing the problem to the limits you know you may assume, in the same manner we have already been doing this.