Assignment 9 - Due Thursday 11/6/2003
Read: Hubbard and Hubbard Section 2.1 and 2.2.
Exercises:
Hand in only the exercises which have stars by them.
Section 2.1 (pages 176-178): 2a*. All of questions 1 - 9 are instructive.
Section 2.2 (pages 185-186): 2e*, 3b*, 6*(b should probably read 'no solution'), 9*, 10*. In questions which ask you to solve a system of equations which has infinitely many solutions, find an expression for the general form of the solution. All of questions 1 - 10 are instructive.
Extra questions:
1. Express the vectors (1,0) and (0,1) as linear combinations of (1,2) and (2,3) by solving an appropriate system of equations for the coefficients of the combinations.
2. Express the vector (5,0,1,2) as a linear combinations of (1,2,1,0) and (2,-1,0,1).
3. In each of the following, determine whether or not the vector v is a linear combiation of the other vectors given:
(a) v = 2i + 3j; a = 2i - j, b = 2i + j.
(b)* v = 2i + 3j + 4k; a = 2i - j, b = i + j + k, c = j - 2k.
(c) v = (3,-1,0,-1); a = (2,-1,3,2), b = (-1,1,1,-3), c = (1,1,9,-5).
4. Solve the systems
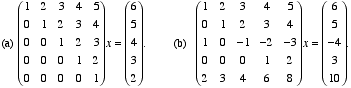
Peter says:
We have an exam on November 6, and hence no quiz. The material which will appear on the exam will be taken from Sections 1.5 - 1.10, starting in Section 1.5 at page 94 where limits, continuity etc. of sequences of vectors and vector-valued functions are introduced. The exam may also test you on material which has appeared in the extra questions on the assignment sheets. You may not use books or notes on the exam, but you may use a calculator.
I hope that we will be able to move quite quickly through the linear algebra of Section 2, as I do not think it is very difficult in principle. I find it irritating that the authors introduce some things which seem to me to make the mathematics seem more complicated than it actually is. The jargon 'pivotal unknowns' is an example of this. It is quite possible to understand what is going on with solving equations by row reduction without knowing what a pivotal unknown is. They comment that Theorem 2.2.4 is practically obvious. I find the difficulty is in working out what on earth they are talking about, not in establishing the result, and the jargon is the difficulty.