Assignment 12 - Due Thursday 4/22/2004
Read: Hubbard and Hubbard Sections 6.7 and 6.8.
Exercises:
Section 6.7 (pages 633-634): 1 - 11, 2*, 8*, 9*.
Section 6.8 (pages 640-642): 1, 2, 3, 5*, 6, 8, 9*, 10*.
Extra Question*: (a) Find a function f(x,y) such that
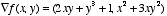 .
.(b) Explain why you cannot find an f(x,y) such that
 .
.Peter's Paragraphs
Once again I feel like rewriting the book. At least this means that you are not seeing the stuff in the book regurgitated when you come to class. (Although it may make you feel like regurgitating.)
I propose not to define the exterior derivative in the way they do in Definition 6.7.1. Instead I think it is more straightforward to define it by means of its algebraic properties, most of which are listed in Theorem 6.7.3. The other two algebraic properties (which we deduce from 6.7.3) are given in 6.7.7 and 6.7.8. The statement of 6.7.1 will then become a theorem, instead of a definition. Almost all the homework exercises you are asked to do are done by applying these algebraic properties.
I think it is not worth troubling too much about the statement of 6.7.1. It is true that I have asked you to do some homework verifying the property of 6.7.1, but perhaps we should not try to understand it too much. When we discuss 6.7.1 I will approach it in the special case of an elementary form and a parallelogram aligned with the coordinate axes. This makes life easier, and the general case is deducible from this.
Something like 6.7.1 is needed for the informal proof of Stokes' theorem which we will do in section 6.9. They do not actually prove Stokes' theorem in that section, and this is a good choice the authors have made, but they do give some idea of why it is true, and we will also discuss this. However, the informal statement about the relationship between the exterior derivative and the boundary of a manifold which I would prefer to use is a little different to 6.7.1. This is why I think we should not trouble ourselves too much with it.
Julian's Joke
Student: What is that you're serving?
Lunch Lady: It's bean casserole.
Student: Yes, but what is it now?