Assignment 3 - Due Thursday 2/12/2004
Read: Hubbard and Hubbard Sections 3.6 and 3.7.
Exercises:
Section 3.6 (pages 359-360): 1, 2*, 6, 7*, 8*.
This book really needs to be proof-read. Question 5 evidently did not get this treatment.
Seciont 3.7 (pages 375-377): 1, 2, 3*, 4*, 5, 6, 7, 8*.
Extra question: A* Let
be defined only on the unit disc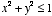 
. Show that on the on the unit disc this function takes its maximum value on the boundary. Calculate the maximum value and a point at which it takes it.Peter's Paragraphs
I specify two sections this week, because I want to have a chance of getting to the end of them in reasonable time. However, Section 3.7 has at the end of it an important piece of linear algebra where eigenvalues and eigenvectors are introduced. There is a lot to be said on this topic, and I am not sure that we will fit it all in, so there are no homework questions specified on this.
Incidentally, I do disagree with their statement that the spectral theorem is probably the most important theorem of linear algebra, at the top of page 372. Of the linear algebra we have done I can think of at least two statements which I consider more important. Of linear algebra we have not done, I consider both the Cayley-Hamilton theorem and the Jordan canonical form more important!
Julian's Joke
What's the difference between broccoli and boogers?
The difference is that kids won't eat broccoli.