Hint for question 1.6.7 on Assignment 5: Read the note in the margin of the book. Consider that function. Where it has a minimum the derivative is zero. Get an expression for that derivative to see that it will produce an answer to the question. You have to show that the minimum cannot occur at either a or b. For this, use the fact that if g is a function for which g'(a) < 0 then for all y in some small open interval (a,a+u) we have g(y) < g(a). The proof of this comes from examining the definition of the limit which appears in defining the derivative.
Assignment 6 - Due Thursday 10/15/2008
Read: Hubbard and Hubbard Section 1.7. My guess is that we might only get up to page 133 or so this week, but let's see!
Exercises:
Hand in only the exercises which have stars by them.
Section 1.7 (pages 142-145): 1d*, 2*, 4b*, 5c*, 7a*, 10*,
Extra Questions
1. For each of the following functions find the directional derivative in the direction of the unit vector u at the point x.

2. Show that the function f defined by
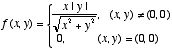
has a directional derivative in every direction at (0,0), but that f is not differentiable at (0,0).
[Hint: If f were differentiable at (0,0) then we would have f'(0,0)=0.]
Comments!:
We have been covering some really difficult stuff, most of which you are seeing in a more sophisticated form than other classes get to see, and which is capable of a still more sophisticated treatment. It is not easy to strike the right balance, and you have been doing really well coming to grips with the proofs and the concepts. Well done!