Topological defect motion in modulated phases
Due to symmetry under rotation, the formation of a modulated phase from an isotropic initial configuration (e.g., a set of parallel rolls in the case of Rayleigh-Benard convection or a lamellar phase of a block copolymer) necessarily results in an ensemble of grains of various orientations, with a large density of grain boundaries and other defects. The longest characteristic relaxation time of such a configuration is directly related to the concentration and spatial distribution of topological defects. Our research focuses on the transient evolution following the decay of a disordered initial configuration and on the motion of various type of defects that control microstructure coarsening. This information will be used to determine characteristic time scales for ordering, as well as to possibly design strategies to speed up the ordering process itself.
This figure shows a typical two dimensional transient configuration with three types of topological defects indicated: dislocations, (+1/2) disclinations and grain boundaries.
An animated temporal sequence of configurations (that includes the figure above) can be seen in this MPEG file (5 MB, calculation by Denis Boyer). Over time the characteristic grain size increases (microstructure coarsening) through a reduction in the density of defects.
Asymptotically close to onset, coarsening is self-similar in that consecutive configurations of the structure are geometrically similar in a statistical sense. The average grain size grows as a power law of time with an exponent of 1/3. This exponent can be understood from the law of grain boundary motion through a background of curved rolls (as seen in the figure and in the animation). This background roll curvature is created and maintained by a distribution of largely immobile disclinations.
At a small but finite distance from onset, the so called non-adiabatic effects appear. They give rise to an effective periodic force on the defects with periodicity twice that of the stripes. Defects now move in a periodic potential and can therefore become pinned when the driving force for motion (related to the curvature of the rolls) falls below a certain threshold related to the amplitude of the periodic potential. An infinite size system will spontaneously evolve into a glassy configuration, with a characteristic grain size which we have estimated as a function of the dimensionless distance from threshold.
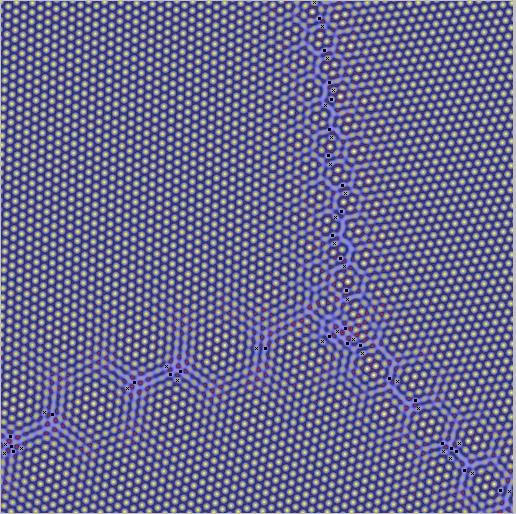The effects of pinning strongly depend on the nature of the
bifurcation that leads to the modulated phase, fact that is related
to the asymptotic width of the defected region as the bifurcation
point is approached. Stripe phases generically bifurcate supercritically
so that the defected region diverges as ε-1/2 when
ε goes to zero. We have shown that pinning effects in this case
become negligible near threshold. Hexagonal phases such as the one shown in
the figure, on the other hand, generically appear subcritically. In this
case, the width of the defect is always finite, and pinning effects on
defect motion cannot be avoided. Therefore, growth and coarsening of
phases that bifurcate subcritically is expected to be slower, and show
a dependence on parameters such as the distance from threshold, or
the amplitude of fluctuations if present.
Current research focuses on incorportaing non variational terms into the equations of defect motion, and reexamining the nature and motion of defects that appear under those circumstamces. Non variational systems (or non gradient, or non potential, as they are also known) are those in which the evolution is not simply governed by free energy minimization. In fact, this is the norm rather than the exception in open, non equilibrium systems for which a generalized free energy may not even exist.